Topology - Tumblr Posts

Math

Geologist: I do more math than you might think

Chemist: I mean, chemical equations are basically mathematical equations. If you think about it (I also do math math)

Physicist: Oh, yeah, it’s all math but we just handwave it

Mathematician: YOU DO WHAT!?

Quantum Physicist: *regularly does math that is literally beyond human comprehension* *now resides in a higher plane of existence*

Engineer: If I don’t do this math correctly PEOPLE WILL DIE

Military Scientist: If I don’t do this math correctly PEOPLE WILL SURVIVE

Topologist: If I don’t do this math correctly PEOPLE WILL BE MOSTLY UNAFFECTED

Philosopher: But what even IS math, really? No seriously, what is it?

Organic Chemist: I kinda forgot how to do math, to be honest

Biologist: I literally only chose this field so I wouldn’t have to do as much math. I love stamp collecting

Biostatistician: wtf

Me duele la cabeza

what is your favorite field of math?

How can you have just one! I'll list a few, in no particular order ^^

Abstract algebra

topology

logic

number theory

probability

Set Theory

The reason for most of these is because of Computer Science

Klein bottle [front and back]

I drew that klein bottle piece back in 2014 or such - and some days ago I inserted it into a foil, and thought it could become nice interactive art: I can insert additional and exchangable foils with patterns and strings into the main foil.

Today's lil möbius strip sketch...

[ballpoint pen]

Math! It's delicious !

I generally adhere to the "traditional" classification of limericks; that is, the poem must be dirty, perverted, somehow sex-related, or simply gross to qualify as a "proper" limerick. However, every once in a while I come across a "clean" limerick that I like so much that I have to make an exception. The anonymous poem below is one of such exceptions.

A mathematician named Klein

Thought the Möbius loop was divine.

Said he: "If you glue

The edges of two,

You'll get a weird bottle like mine."

If you are not familiar with the concept Klein bottles or Möbius loops, I highly suggest utilizing Google and/or Wikipedia as they are quite weird and a lot of fun (and you can easily make yourself a Möbius loop to play with!).

For further reading: Acme Klein Bottles has a whole page of Klein bottle cartoons and limericks. It's awesome; you should check it out.

I will formally conclude this post with some spiffy Möbius loop/Klein bottle animation.

Möbius loop

Klein bottle

Klein bottle with highlighted Möbius loop

And finally, a Klein bottle hat that I might just have to order for myself...

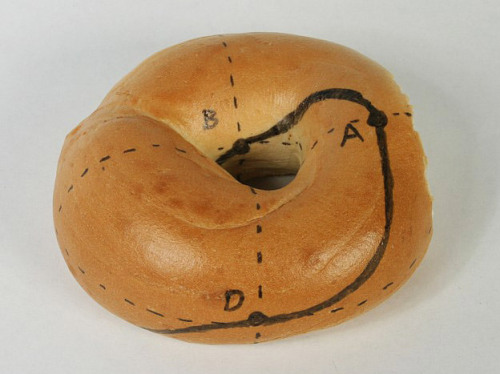
Science-ify your breakfast with a Möbius bagel

You’ve probably heard of a Möbius strip before - it’s a continuous shape that only has one side and one edge. You can make one pretty easily by cutting a strip of paper, giving it a half twist, and taping the ends together to form a loop. However, if you want to really impress, make a Möbius bagel. By following the instructions, you can cut your bagel (or your donut!) into two interlocking bagel halves. From now on, eat your breakfast like a Scientist!

no roboclock yet, but we did print a klein bottle

[dl the thing]

This isn't quite true; this is non-Euclidean geometry, and basically they said "parallel lines always stay a certain distance away? well, we can take that in 2 directions: either they always meet, or they always diverge!" and then we got elliptic geometry (ex. surface of the earth) and hyperbolic geometry (imagine taking a pringle or saddle and extending the ends really far), which are really cool!

Topology studies how we can deform certain shapes in different ways and it's really cool! You may have seen the coffee mug turning into a donut, but topology also studies the difference between knots and circles, donuts and donut holes, Möbius strips and tubes, etc! It's pretty advanced math once you get further, but the basics are easy to understand.

Me duele la cabeza

I’d like to share a couple of highlights of my discord messages today.

7:42 pm

AAAAAAA I HATE INDUCTION

The book my team is using as a reference suppressed a really specific detail of a proof by hiding it as an exercise

And it’s like 8 pages of induction

11:40 pm

Frothing at the mouth ok there are a couple of problems with my induction and the actual proof is like three lines of triangle inequality.

anyway, the moral of the story is that in a δ-hyperbolic geodesic space (one where any point on a side of a triangle is within δ of the other two sides), any 8δ-local geodesic (a path that is preserves distances between any two points within 8δ of each other in the domain) stays uniformly within 2δ of the geodesic connecting its endpoints.

Closed is not the opposite of Open. Closed is the complement of Open. Ifykyk

A surface bounded by four interlocking "triangular" loops, made with Shiying Dong's seamless topological crochet method